I give talks on a variety of mathematical topics regularly. I’ve enjoyed talking to all audiences: large KS4 and A-level enrichment days, at the Royal Institution, at academic colloquia, and for a pop-science adult audience at Science Showoff and the Boring Talks.
The talks listed here are accessible to general and scientific audiences. For academic talks on my research, see my research page.
If you’d like me to talk at your event, drop me an email.
Contents
Talks for everyone
The maths of chocolate fountains

Image: LMS
Although adapted to fit the audience, everything I present here comes from my master’s project, which was published in a research journal in 2015, so it’s genuine applied maths research! And I always bring the fountain to every talk. Topics brought in include:

Image: Luciano Rila
- good and bad models for everyday things (and how to tell between them),
- how mayonnaise and cornflour paste react in opposite ways when hit,
- the link between these liquids and the graphs of $y = x^n$ for different $n$,
- why teapots drip backwards (and how we could fix this).
Good for all ages, as I showcase the scientific method. GCSE students enjoy seeing the power of the power law. For A-level students, I reveal the secrets of fluid mechanics, and show why it’s harder than the mechanics they see at A-level.
Works best as an hour-long talk, with some time for eating chocolate afterwards.
- The Washington Post: Someone finally looked into the physics of chocolate fountains
- Daily Mail: The physics of a chocolate fountain: Curtains of melted goo pull inwards because of surface tension
- Smithsonian Institution: Chocolate fountains are great for physics lessons
- Heart Breakfast with Ed & Rachel: Chocolate? It’s Good But What Else Can We Fountain?
- Read about the maths of chocolate fountains research project
Making coins better: a call for change

- which country has the heaviest coins,
- how many prices actually end in 99p these days,
- which new coin the UK should add to reduce the weight in our pockets.
Works best as a 20-minute talk.
- Boring Talks on BBC Sounds: Change (17 min)
- Chalkdust: Forget a new £1 coin, we need a £1.23 coin*
- Chalkdust: Why self-service machines give such awful change
* With the latest ONS data, I have reconsidered this number…
Is there a perfect maths font?
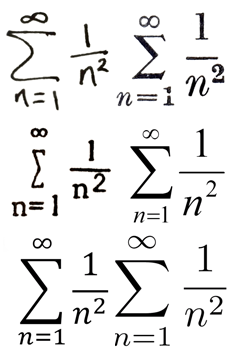
You’ve spent hours, perhaps days, on this one piece of maths, and finally it’s finished. It’s elegant and beautiful. But now you need to type and print it, and there’s a question you face: what font should I choose?
Based on my popular article, I give a history of typesetting mathematics, and why it really does matter which font you pick. Discover:
- which font is the most trustworthy,
- why maths typesetting is really so hard,
- why getting the fonts right really matters in mathematics.
Works best at 30 minutes.
- Chalkdust: Is there a perfect maths font?
Talks for new PhD students
Things I wish I knew about scientific computing before I started scientific computing
Shortly after starting my maths PhD, I came up against a numerical problem that required writing some code. I had some programming experience (as a hobby, making daft websites and that sort of thing) but was surprised at how little of it was transferrable to computing specifically for science.
In the ten years since, as I’ve worked my way through a bunch of scientific computing problems related mostly to fluid dynamics, I’ve picked up a bunch of tips which I think I would have liked to have known from the beginning. This talk modestly aims to fill that gap!
Although I am an applied mathematician, this talk should be accessible to everyone across the mathematics spectrum, and will include honest reflections on a bunch of tools that the audience might be using, or might want to use.
For the novice, I hope to provide a broad sense of the landscape and a bunch of tips on where to start. For the expert, I hope to provide little bits of experience which boosted my productivity and might boost yours.
Talks for A-level students
The maths of music
Enjoying or making music is a wonderful human pastime. It is no surprise then, that mathematicians have dug into it to try and find some meaning within the art.
This talk combines music and physics with a simple introduction to group theory. We start at simple transpositions and end up seeing Beethoven journey round a torus. I bring along a mini keyboard to demonstrate:
- how songs from today share the same mathematical structure as songs from the 50s,
- what the Pythagoreans already knew about music,
- what music sounds like when you go between the notes.
Suitable from GCSE, but is best appreciated by A-level onwards. Works best as a 40-minute talk. Also exists in an undergraduate mathematics version.
Using maths to pass your driving test
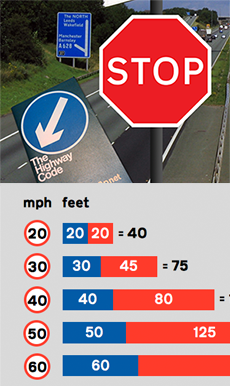
Image: Klaus with K, CC-BY-SA 3.0; Paul Downey, CC-BY 2.0
Passing your driving test requires practising various manoeuvres and memorising things like stopping distances. But memorising is boring! With just the smallest amount of mechanics, you can figure out some useful equations that govern the data given in the Highway Code. But is this data actually any good? Based on an article I wrote, find out:
- how the units you choose have a big impact on how memorable these formulas are,
- whether lorries brake faster than cars,
- why British people seem to believe that they have much faster reactions than citizens of any other country.
Suitable for GCSE upwards, but particularly nice for A-level students who are beginning to learn to drive, as well as doing mechanics in their maths A-level.
Works best at 30 minutes.
- Chalkdust: Stopping distances in the Highway Code are wrong
The problem solving workshop

Take a large slab of chocolate and try to snap it into all its little pieces. How many snaps do you need? What if it’s not rectangular? What if it has a hole in the middle?
The art of problem solving is ripe for discussion but in this workshop we look at a few techniques that are worth remembering when first looking at mathematical problems, including:
- taking things to their extremes,
- looking for symmetry,
- the value of guessing!
We also discuss the value of good mathematical communication, and how to make a convincing mathematical argument.
Suitable for A-level or late GCSE. Works as an interactive workshop with students working in small groups, for around two hours.
Workshops for GCSE students
The coins-in-a-square workshop

What’s the smallest square that fits around three identical coins? What about 4? 9? 100? What pattern do you need to arrange the coins in, in order to fit in that smallest square? And then how much of the square is covered with coins, in each case?
In this interactive workshop, students are given the opportunity to play around and try to work these questions out for themselves. Can they form conjectures about the pattern you need to use for square numbers of coins, for example?
We talk about:
- how airlines can use this tactic to make flying worse,
- what train announcers should be telling passengers to do to get more people on the train,
- how efficiency in business is a mathematical problem.
Works best as a 30-minute workshop.
The modelling workshop

Suppose someone comes up to you and asks you to describe some interesting behaviour mathematically. Where do you start?
This interactive workshop builds the confidence of students to just get out their pencils and try stuff! Can they derive Newton’s law of gravitation just by intuition? Then how can they test it out? We look at models they may encounter later in physics or economics, and get students to discuss in which ways their models are better or worse than their classmates’.
We also look at mathematics and models in the newspapers and social media. Can we tell a good model from a bad model? Or, in an era of fake news, is the model fine, and the journalism bad?
Works best as a 30-minute workshop.
